Factoring Polynomials

Page
2

Find the GCF of the following list of terms.

a3b2, a2b5 and a4b7

a3b2 = a · a · a · b · b

a2b5 = a · a · b · b · b · b · b

a4b7 = a · a · a · a · b · b · b · b · b · b · b

So the GCF is a · a · b · b = a2b2

Notice that the GCF of terms containing variables will use the smallest exponent found amongst the individual terms for each variable.

Greatest Common Factor

Example

Slide 10

Factoring Polynomials

The first step in factoring a polynomial is to find the GCF of all its terms.

Then we write the polynomial as a product by factoring out the GCF from all the terms.

The remaining factors in each term will form a polynomial.

Slide 11

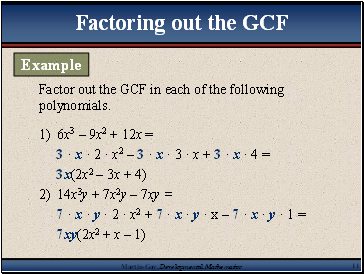
Factoring out the GCF

Factor out the GCF in each of the following polynomials.

1) 6x3 – 9x2 + 12x =

3 · x · 2 · x2 – 3 · x · 3 · x + 3 · x · 4 =

3x(2x2 – 3x + 4)

2) 14x3y + 7x2y – 7xy =

7 · x · y · 2 · x2 + 7 · x · y · x – 7 · x · y · 1 =

7xy(2x2 + x – 1)

Example

Slide 12

Factor out the GCF in each of the following polynomials.

1) 6(x + 2) – y(x + 2) =

6 · (x + 2) – y · (x + 2) =

(x + 2)(6 – y)

2) xy(y + 1) – (y + 1) =

xy · (y + 1) – 1 · (y + 1) =

(y + 1)(xy – 1)

Factoring out the GCF

Example

Slide 13

Factoring

Remember that factoring out the GCF from the terms of a polynomial should always be the first step in factoring a polynomial.

This will usually be followed by additional steps in the process.

Factor 90 + 15y2 – 18x – 3xy2.

90 + 15y2 – 18x – 3xy2 = 3(30 + 5y2 – 6x – xy2) =

3(5 · 6 + 5 · y2 – 6 · x – x · y2) =

3(5(6 + y2) – x (6 + y2)) =

3(6 + y2)(5 – x)

Example

Slide 14

§ 13.2

Factoring Trinomials of the Form x2 + bx + c

Slide 15

Factoring Trinomials

Recall by using the FOIL method that

F O I L

(x + 2)(x + 4) = x2 + 4x + 2x + 8

= x2 + 6x + 8

To factor x2 + bx + c into (x + one #)(x + another #), note that b is the sum of the two numbers and c is the product of the two numbers.

So we’ll be looking for 2 numbers whose product is c and whose sum is b.

Note: there are fewer choices for the product, so that’s why we start there first.

Slide 16

Factoring Polynomials

Factor the polynomial x2 + 13x + 30.

Since our two numbers must have a product of 30 and a sum of 13, the two numbers must both be positive.