Antiderivatives, Differential Equations, and Slope Fields

Page
1

Slide 1

AP Calculus AB

Antiderivatives,

Differential Equations,

and Slope Fields

Slide 2

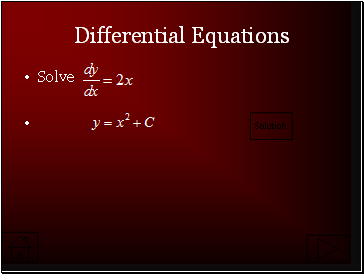
Review

Solution

Consider the equation

Slide 3

Antiderivatives

What is an inverse operation?

Examples include:

Addition and subtraction

Multiplication and division

Exponents and logariths

Slide 4

Antiderivatives

Differentiation also has an inverse…

antidefferentiation

Slide 5

Antiderivatives

Consider the function whose derivative is given by .

What is ?

Solution

We say that is an antiderivative of .

Slide 6

Antiderivatives

Notice that we say is an antiderivative and not the antiderivative. Why?

Since is an antiderivative of , we can

say that .

If and , find

and .

Slide 7

Differential Equations

Recall the earlier equation .

This is called a differential equation and could also be written as .

We can think of solving a differential equation as being similar to solving any other equation.

Slide 8

Differential Equations

Trying to find y as a function of x

Can only find indefinite solutions

Slide 9

Differential Equations

There are two basic steps to follow:

1. Isolate the differential

Invert both sides…in other words, find

the antiderivative

Slide 10

Differential Equations

Since we are only finding indefinite solutions, we must indicate the ambiguity of the constant.

Normally, this is done through using a letter to represent any constant. Generally, we use C.

Slide 11

Solution

Differential Equations

Solve

Slide 12

Slope Fields

Consider the following:

HippoCampus

Slide 13

Slope Fields

A slope field shows the general “flow” of a differential equation’s solution.